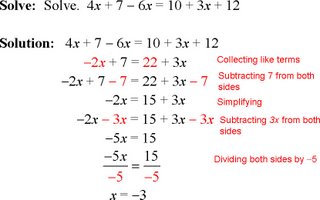
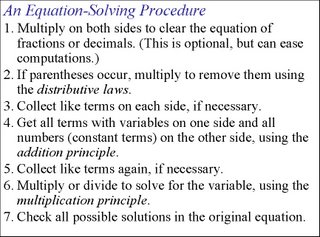
Solving Equations and Inequalities 2.7

2.7 SOLVING INEQUALITIES
a. Determine whether a given number is a solution of an inequality.
b. Graph an inequality on a number line.
c. Solve inequalities using the addition principle.
d. Solve inequalities using the multiplication principle.
e. Solve inequalities using the addition and multiplication principles together.

Objective a
Determine whether a given number is a solution of an inequality.



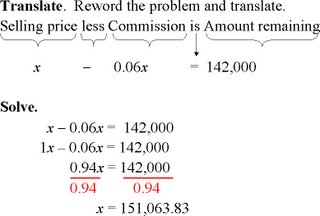
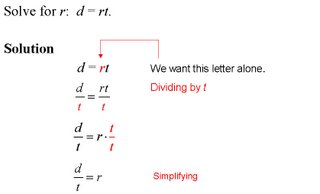
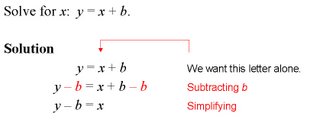
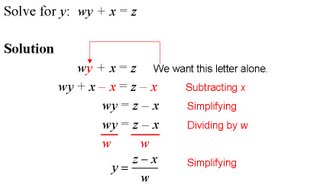
Example A



Objective b
Graph an inequality on a number line.

Graphs of Inequalities
Because solutions of inequalities like x < 4 are too numerous to list, it is helpful to make a drawing that represents all the solutions.
The graph of an inequality is a drawing that represents its solutions. Graphs of inequalities in one variable can be drawn on a number line by shading all the points that are solutions. Open dots are used to indicate endpoints that are not solutions and closed dots are used to indicated endpoints that are solutions.

Example B




Objective c
Solve inequalities using the addition principle.



Example C



Example D



Objective d
Solve inequalities using the multiplication principle.



Example E




Objective e
Solve inequalities using the addition and multiplication principles together.

Example F



Example G



Example H

Solving Equations and Inequalities 2.6

2.6 APPLICATIONS AND PROBLEM SOLVING
a. Solve applied problems by translating to equations.

Objective a
Solve applied problems by translating to equations.

Five Steps for Problem Solving in Algebra
1. Familiarize yourself with the problem situation.
2. Translate the problem to an equation.
3. Solve the equation.
4. Check the answer in the original problem.
5. State the answer to the problem clearly.




Example A




Example B






Example C





Example D




Example E

Solving Equations and Inequalities 2.5

2.5 Applications of Percent
a. Solve applied problems involving percent.

Objective a
Solve applied problems involving percent.





Example A



Example B



Example C



In solving percent problems, we use the Translate and Solve steps in the problem-solving strategy used throughout the text.





Example D: Finding the Amount



Example E: Finding the Base



Example F: Finding the Percent



Example G



Example H

Solving Equations and Inequalities 2.4

2.4 Formulas
a. Evaluate a formula.
b. Solve a formula for a specified variable.

Objective a
Evaluate a formula.

Many applications of mathematics involve relationships among two or more quantities. An equation that represents such a relationship will use two or more letters and is known as a formula.

Example A



Objective b
Solve a formula for a specified variable.

Example B



Example C



Example D



To solve a formula for a given letter, identify the letter and:
1. Multiply on both sides to clear fractions or decimals, if that is needed.
2. Collect like terms on each side, if necessary.
3. Get all terms with the letter to be solved for on one side of the equation and all other terms on the other side.
4. Collect like terms again, if necessary.
5. Solve for the letter in question.

Example E

Solving Equations and Inequalities 2.3

2.3 USING THE PRINCIPLES TOGETHER
a. Solve equations using both the addition and multiplication principles.
b. Solve equations in which like terms need to be collected.
c. Solve equations by first removing parentheses and collecting like terms; solve equations with no solutions and equations with an infinite number of solutions.

Objective a
Solve equations using both the addition and multiplication principles.

Example A



Example B



Objective b
Solve equations in which like terms need to be collected.

Combining Like Terms
If like terms appear on the same side of an equation, we combine them and then solve.

Should like terms appear on both sides of an equation, we can use the addition principle to rewrite all like terms on one side.

Example C



Example D



Clearing Fractions and Decimals
In general, equations are easier to solve if they do not contain fractions or decimals.

The easiest way to clear an equation of fractions is to multiply every term on both sides by the least common multiple of all the denominators.

To clear an equation of decimals, we count the greatest number of decimals places in any one number and multiply on both sides by that multiple of 10.

Example E



Objective c
Solve equations by first removing parentheses and collecting like terms; solve equations with no solutions and equations with an infinite number of solutions.

Example F





Example G



Example H



Example I