Solving Equations and Inequalities 2.3

2.3 USING THE PRINCIPLES TOGETHER
a. Solve equations using both the addition and multiplication principles.
b. Solve equations in which like terms need to be collected.
c. Solve equations by first removing parentheses and collecting like terms; solve equations with no solutions and equations with an infinite number of solutions.

Objective a
Solve equations using both the addition and multiplication principles.

Example A



Example B



Objective b
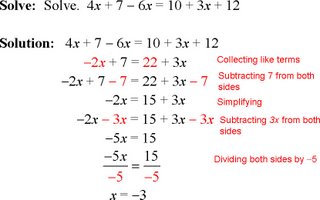
Solve equations in which like terms need to be collected.

Combining Like Terms
If like terms appear on the same side of an equation, we combine them and then solve.

Should like terms appear on both sides of an equation, we can use the addition principle to rewrite all like terms on one side.

Example C



Example D



Clearing Fractions and Decimals
In general, equations are easier to solve if they do not contain fractions or decimals.

The easiest way to clear an equation of fractions is to multiply every term on both sides by the least common multiple of all the denominators.

To clear an equation of decimals, we count the greatest number of decimals places in any one number and multiply on both sides by that multiple of 10.

Example E



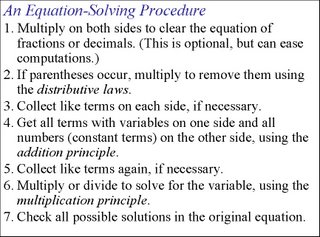
Objective c
Solve equations by first removing parentheses and collecting like terms; solve equations with no solutions and equations with an infinite number of solutions.

Example F





Example G



Example H



Example I