Introduction to Real Numbers and Algebraic Expressions 1.8

1.8 SIMPLIFYING EXPRESSION; ORDER OF OPERATIONS
a. Find an equivalent expression for an opposite without parentheses, where an expression has several terms.
b. Simplify expressions by removing parentheses and collecting like terms.
c. Simplify expressions with parentheses inside parentheses.
d. Simplify expressions using rules from order of operations.

Objective a
Find an equivalent expression for an opposite without parentheses, where an expression has several terms.



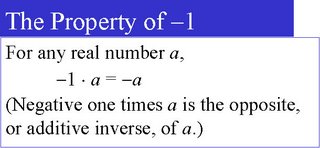
Example A



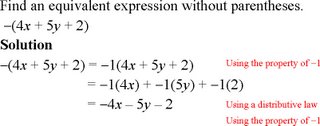
Example B



Objective b
Simplify expressions by removing parentheses and collecting like terms.

Example C



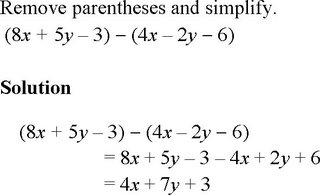
Example D



Objective c
Simplify expressions with parentheses inside parentheses.

When more than one kind of grouping symbol occurs, do the computations in the innermost ones first. Then work from the inside out.

Example E





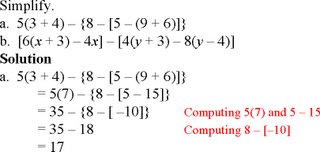
Objective d
Simplify expressions using the rules for order of operations.

Rules for Order of Operations
1. Do all calculations within grouping symbols before operations outside.
2. Evaluate all exponential expressions.
3. Do all multiplications and divisions in order from left to right.
4. Do all additions and subtractions in order from left to right.

Example F



Example G